This is a continuation of a test of the following lenses on the Sony a7RII:
- Zeiss 85mm f/1.8 Batis.
- Zeiss 85mm f/1.4 Otus.
- Leica 90mm f/2 Apo Summicron-M ASPH.
- AF-S Nikkor 85mm f/1.4 G.
- Sony 90mm f/2.8 FE Macro.
The test starts here.
This posts repeats the testing regime of the previous one with a different lens, the Leica Apo-Summicron. The term Apo as applied to a lens does not, unfortunately, have a precise meaning. In general, it means that the lens is corrected for three wavelengths of light, but there is no universal specification of what those wave lengths should be (550, 551, and 552 nm, anybody?) and how good the correction has to be. This test doesn’t use single wavelengths anyway, but rather groups of them that are determined by the camera’s spectral response, the lens itself, the target reflectivity, and the lighting. In general, the designation Apo on a lens means that the folks in marketing think that the lens is well corrected by their company’s standards, and that they would like you to think that, too.
How well-corrected is the ‘cron for LoCA? We shall see.
I lit the target with two Westcott LED panels, and set the color temperature to 5000K. I mounted a Sony a7RII with an Apo- Summicron on it to the Cognisys computer-driven focusing rail. I set the controller up to use 192mm of travel and make 49 exposures 4mm apart. I set the assembly 3.3 meters from the on-axis target, mounted the lens, focused a little towards the front from mid-rail using manual focusing, and exposed at 49-shot series from f/2 through f/8. I used Jack Hogan’s Matlab program, MTF Mapper, and DCRAW to calculate the MTF50s for the raw color planes, imported the data into Excel, and plotted the results in cycles per picture height vs image distance change. Earlier I showed curves that were referenced to subject distance, but I’m changing over to measuring focus shift at the sensor so as to be able to more fairly compare LoCA performance of lenses of different focal length.
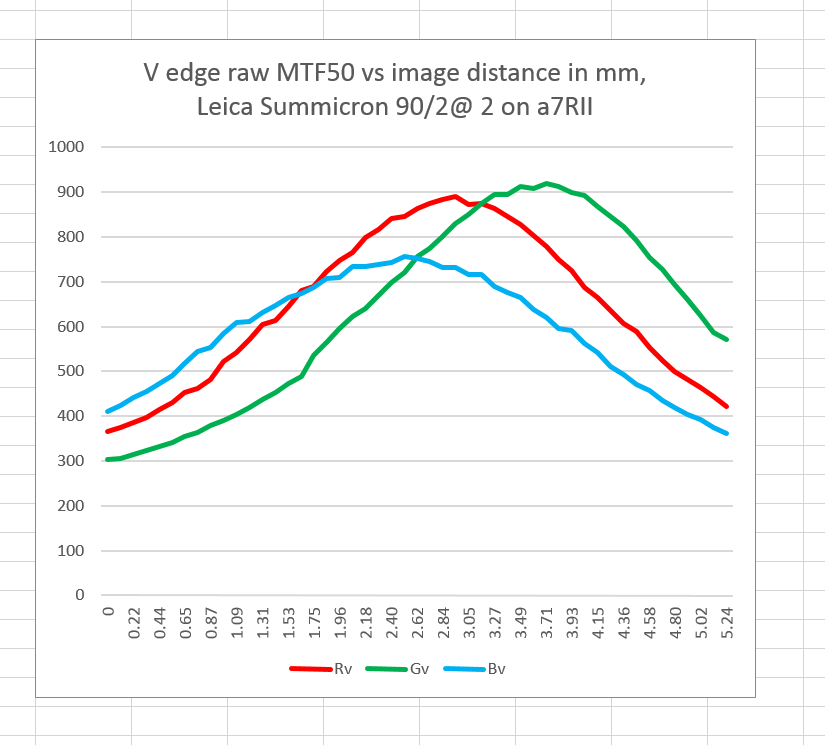
At f/2:
The top set of curves is for the horizontal edge. The bottom is for the vertical edge. There is a slight bit of astigmatism as shown by the difference in the position of the peaks of the two curves, but nothing that would concern anyone in actual use of the lens. In the Batis, we saw the green and the blue planes being focused in the same location. Not so here; the green and blue are the furthest apart. The spread between the three planes is a bit over 3 mm, somewhat less that for the Batis at a the same f-stop.
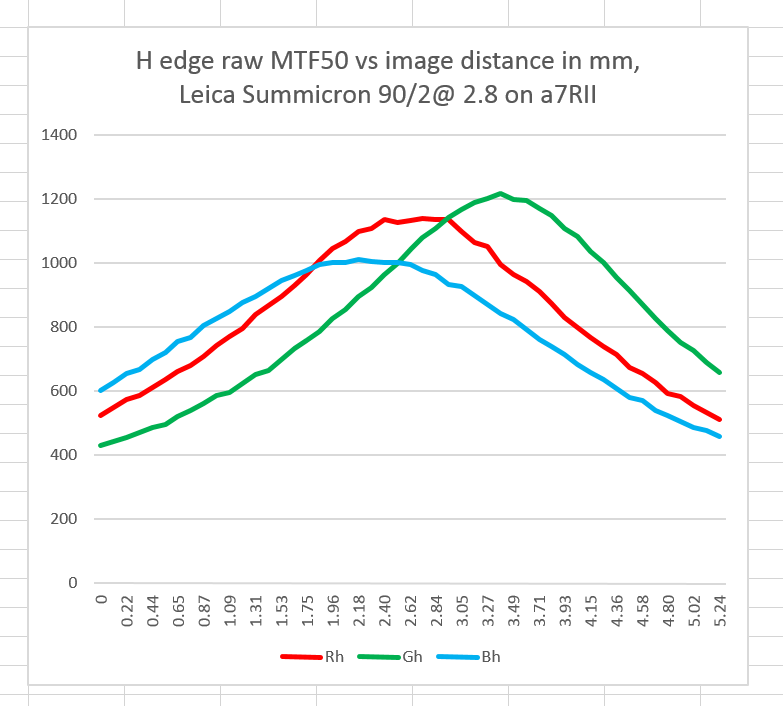
At f/2.8:
Not much has changed, except for focus shift.
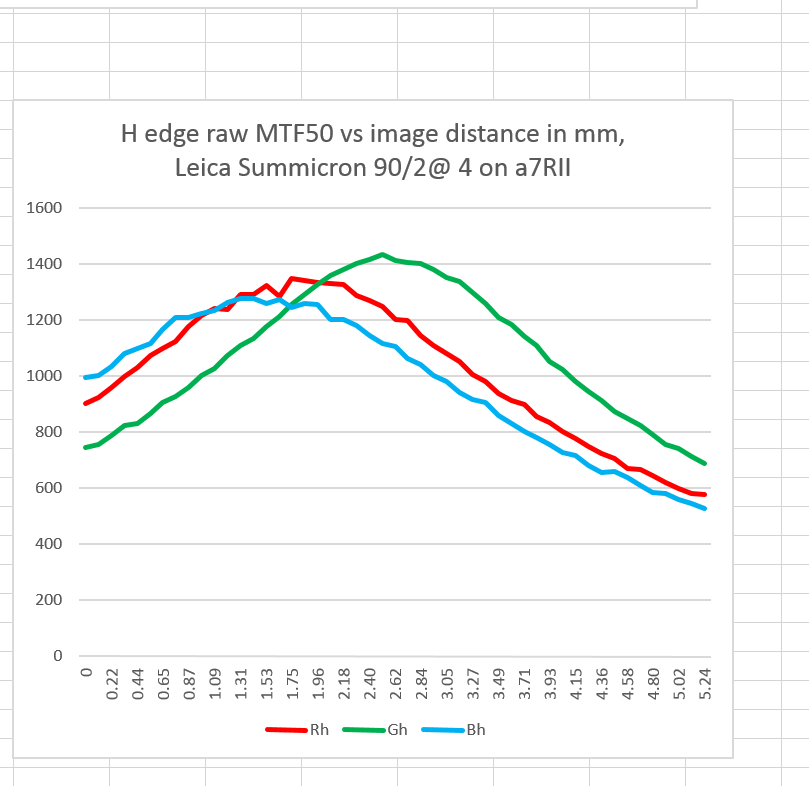
At f/4:
Now the peaks are coming closer together.
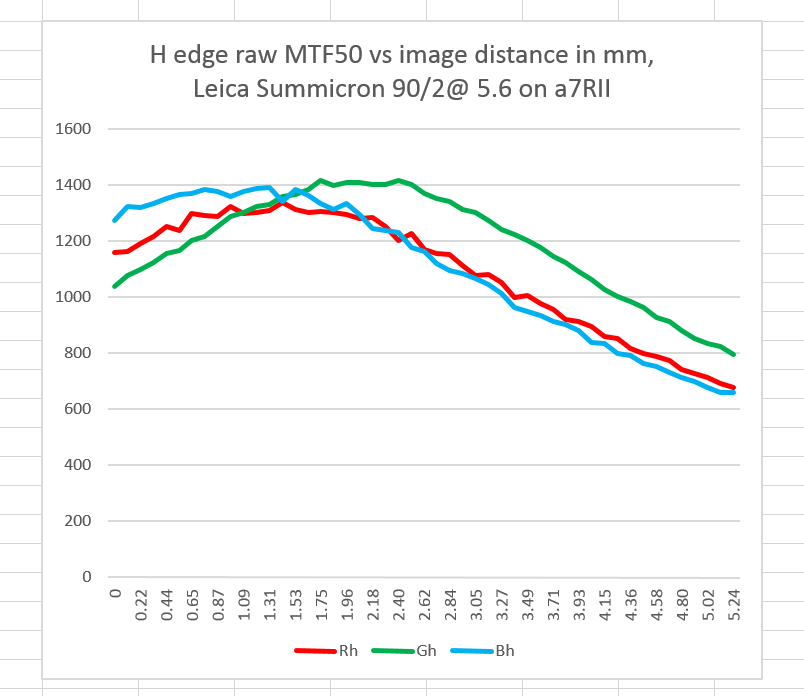
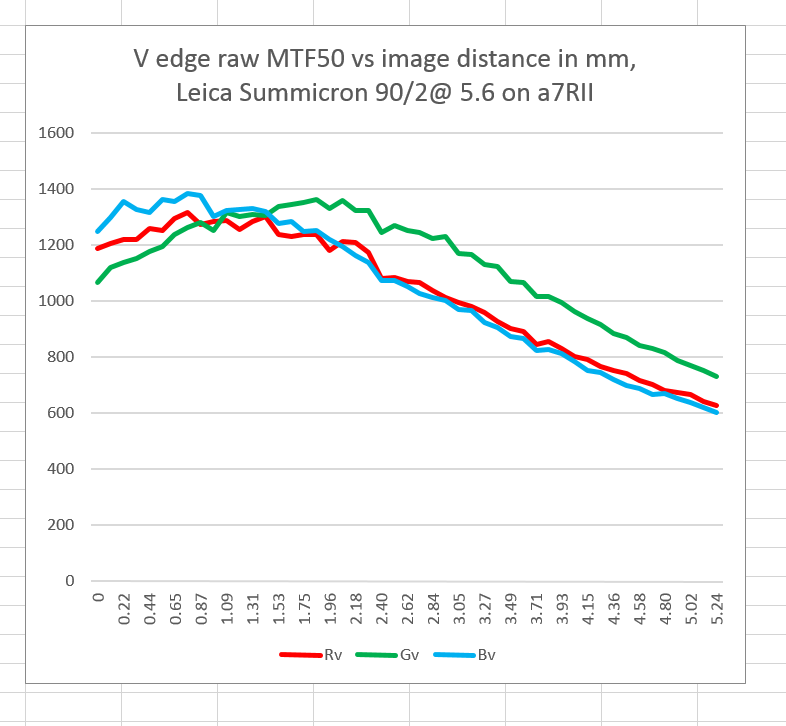
At f/5.6:
The red and blue planes are almost identical, and the green is slightly shifted.
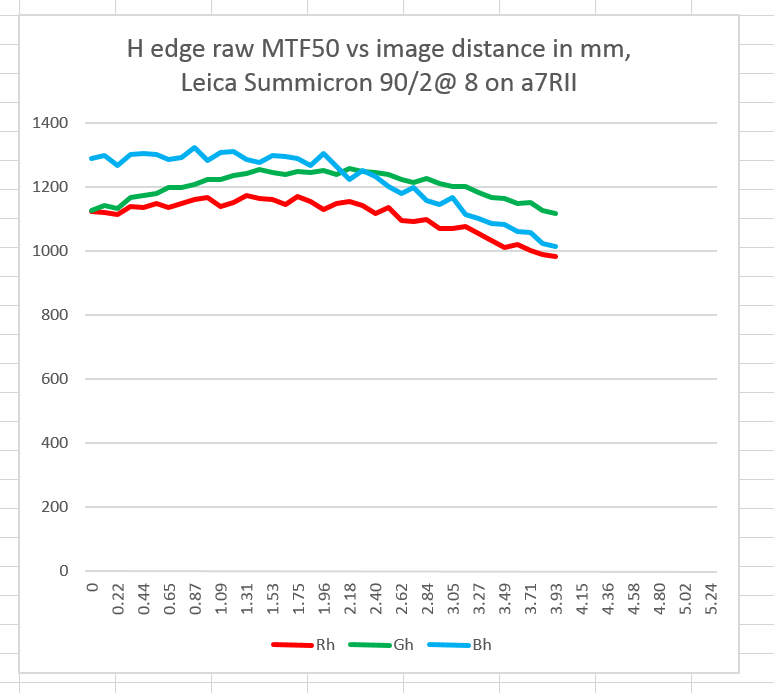
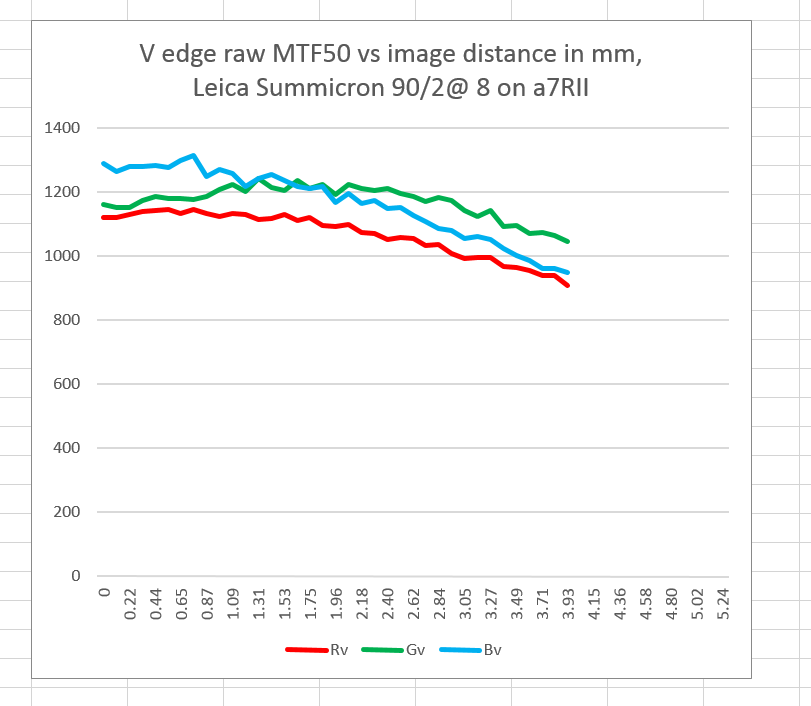
And finally, at f/8:
The increase depth of field has swamped out the LoCA. The reason why the curves don’t do all the way across the graph is that the Cognisys rail had a hiccup and aborted the operation. It seems to do that about one exposure in 1000, which is too infrequent to troubleshoot, but not too infrequent to be a PITA. Fortunately this time it happened on the last run of the series.



Looks better corrected than the Batis.
I’ve noticed that different lenses have different LoCA color fringes: most standard lenses are magenta/green, where red and blue hang together more, and others (apparently including the Batis) are red/cyan where blue and green match more closely. A notable example of the latter is the Samyang 35/1.4.
Personally I prefer the look of lenses with more green/magenta if there is to be any fringing at all, but that may be just what I’m used to.
So where exactly in these charts is a lens ‘best focus’? Considering the photopic curve and relative number of cones I would guess when the green channel hits a peak.
Jack
If best focus is the peak of the luminance curve, then best focus would be a little bit away from the peak of the green curve in the red direction. It’s hard to say, since we’re not looking at planes that have any colorimetric meaning, although we call then red. blue, and green. They’re just raw planes.