Yesterday, I presented the result of fitting the a7II data to the standard photon-transfer model. Today I’ll compare those results to two other cameras, the Nikon D4 and D810.
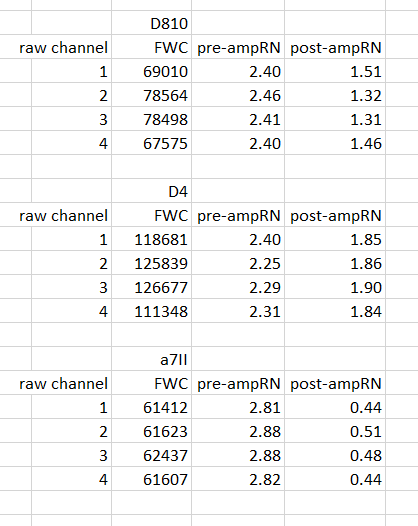
Here are the numbers:
In the table above, we separate the read noise into two components, as described earlier. The first is the read noise on the sensor side of the amplifier whose gain is controlled by the ISO knob; that’s the pre-amp read noise, and its units are electrons. The second is the read noise on the ADC side of the amplifier. I call that the post-amp read noise, and its units are ADC LSBs. Let me explain that last unit a bit. Yes, the post-amp read noise is an analog quantity, and we could measure it in volts — actually microvolts — but that wouldn’t mean much to us as photographers. We care about how it makes the digitized result uncertain, and thus it is natural to measure it in the units that we see when we look at the raw files.
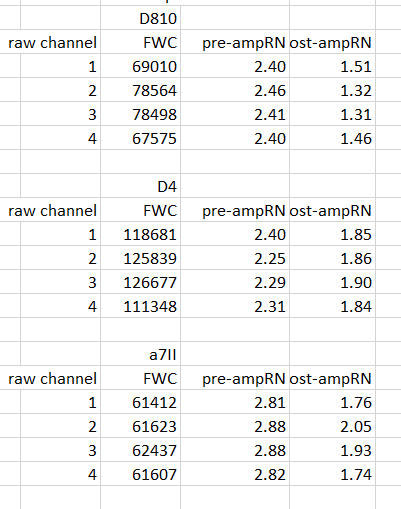
Correcting for the fact that the two Nikons are 14-bit cameras, and the Sony is, for the purpose of this discussion a 12=bit one:
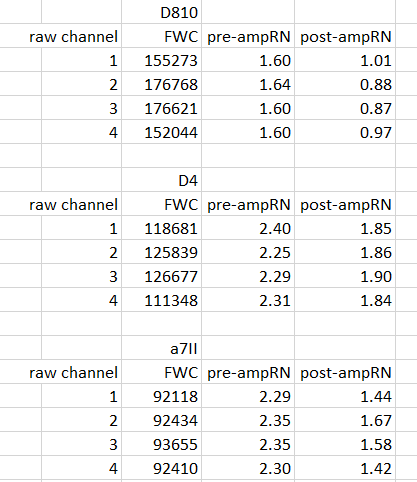
All three cameras have full frame sensors, but the pixel pitch and therefor the resolution differ. To make the comparison apples and apples, I multiplied the FWC of the Sony by 24/16 = 1.5, and the FWC of the D810 by 36/16 = 2.25. I multiplied the two RN values of the Sony by sqrt(16/24), and the two RN values of the D810 by sqrt(16/36), to simulate the noise reduction you’d see with perfect down-sampling to the same pixel count.
The D810 stands out as a pretty remarkable camera. I didn’t consider the fact that the D810 gives away two-thirds of a stop of light-gathering power, since its base ISO is 64 and the other two cameras have a base ISO of 100. I don’t know how to factor that in. I’d love to get my hands on a D4s for a set of test images, but not enough to shell out the dough.
Leave a Reply