The intellectual underpinnings of MTF analysis for handheld images, part 2

Actually, this post applies to MTF analysis in general, whether the camera is on a tripod or not.

In the previous post, I said:

to get the system’s modulation transfer function (MTF), we perform a Fourier transform on the system’s impulse response, or point-spread function (PSF), and throw away the imaginary part.

What do we give up by ignoring the imaginary, or phase, part of the Fourier transform of the PSF? Plenty. Without the phase part, we don’t have enough information to get back to the PSF. In fact, without the phase part, there are an infinite number of PSF’s that could have any given MTF.

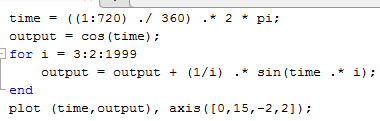
Let me give you a concrete, one-dimensional example. I wrote this little Matlab script to compute the first five terms of the Fourier series for a square wave:

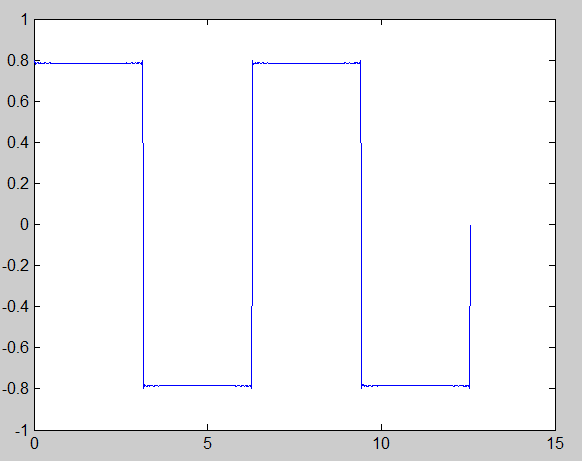
When you run the code, this is what you get:

Note the cute little Gibb’s Ears of overshoot and pre-shoot. They go away as the number of terms approaches infinity.

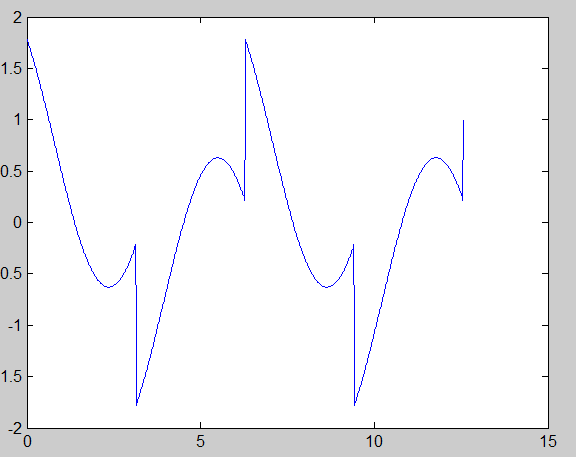
Now, let’s make one teensy three-letter change to the code, so that the fundamental frequency is 90 degrees out of phase with all the others:

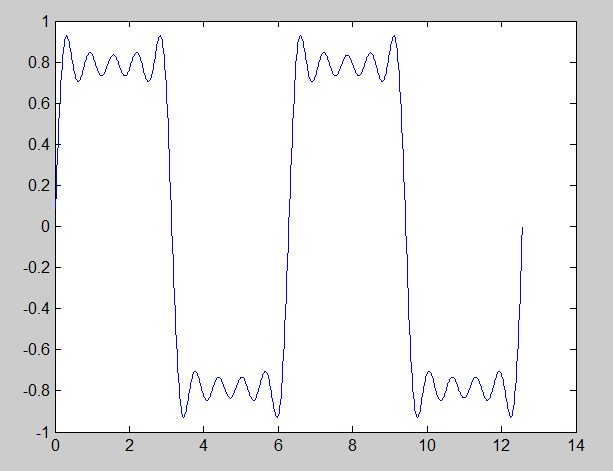
When we run this code, here’s what we get:

Oops, it doesn’t look anything like a square wave, does it?

The difference is even more striking if we use the first 1000 terms.

The code:

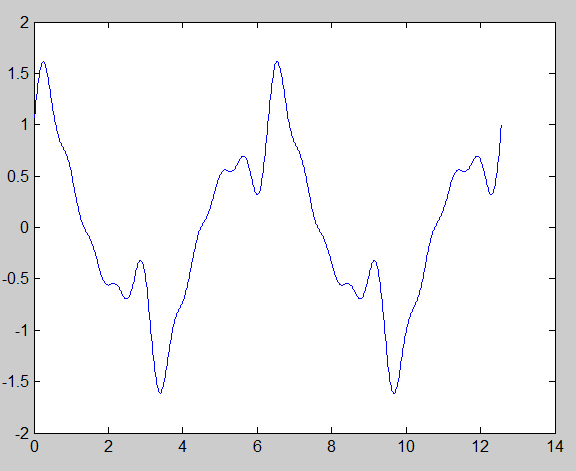
The result:

Changing the phase of the first term by 90 degrees:

The result:

Throwing away the phase part is a common thing for engineers to do. It makes the data a lot more tractable, the graphs easier to read, and, in general, makes life more pleasant. However, it can lead to big mistakes. When the Compact Disc first came out, there was something wrong with the way the high frequencies sounded. The engineers had designed the anti-aliasing filters by looking at just the amplitude part of the filter transfer function, and the phase part had wild variations. The engineers argued that people couldn’t hear phase. They were wrong, as anyone with three function generators, a summing amp, and a pair of headphones can demonstrate to themselves. Modern CD players and recorders use oversampling and AA filters that consider phase.

So as we blithely sling around those MTF curves, we should remember that something is missing.