This is a continuation of a series of posts about adapter pitfalls and pleasures (I promise that I’ll get to the pleasures). The first two posts are here and here.
It has been stated by many that adapter tilt affects focus blur more for short lenses than long ones. It’s become conventional wisdom, but it has never sat right with me.
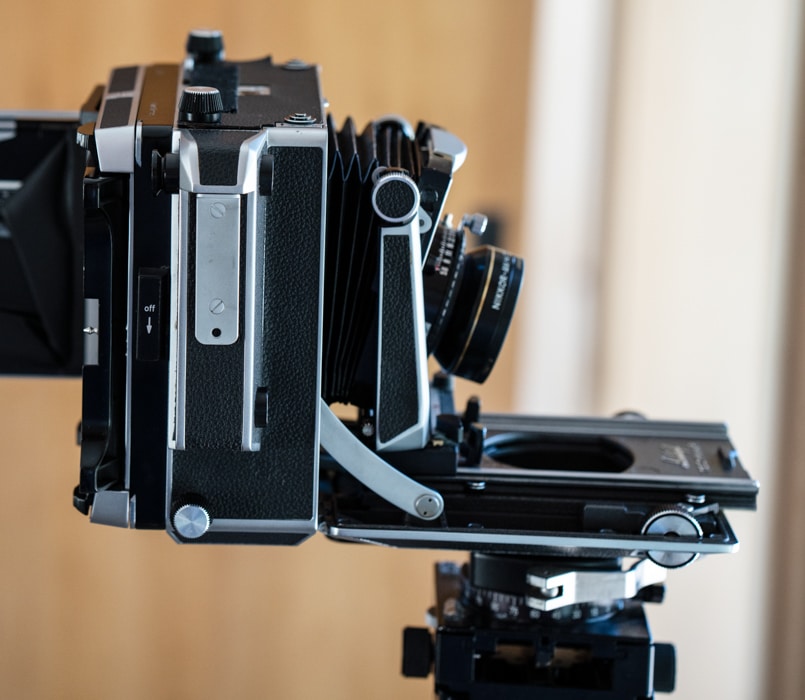
I constructed an actual physical experiment. I took a Linhof Master Technika, and two lenses, a Nikkor SW 65 mm f/4 and a Nikkor AM-ED 120 mm f/5.6. I printed out a checkerboard target and applied a boatload of tilt — I almost used another term, but this is a family-friendly blog. This picture will give you a rough idea, even if the tilt wasn’t quite the same for the checkerboard shots:
I set the camera up with all the movements but tilt centered, and square on to the target. I slipped a Betterlight Super 6K back into the film holder, and lit the target like this:
Then I spent 2 hours trying to get the back to run under Windows 10 — the last time I’d used it was about 3 years ago, and I was using an XP laptop, since sold. I couldn’t find a USB driver that worked and gave up; I’m going to sell the back.
But I didn’t give up and ended up taking pictures of the ground glass. It ain’t pretty, but I think it proves the point.
Here’s the 120 at indicated f/5.6. The effective aperture is maybe a third of a stop narrower than that because of bellows extension.
As expected, both the top and the bottom are out of focus, and the center is sharp.
Here’s the 65 at the same f-stop, the same reproduction ratio, and therefore the same effective f-stop:
Because it’s such a short lens, there’s a lot of light fall-off. I tried to even it out:
Now you can see clearly that there is more blur at the top and bottom of the image made with the shorter lens. It’s possible that at the same distance the two lenses would have similar blur, but I think the right way to look at the situation is to hold the magnification constant.
Score: one for reality, one for conventional wisdom, and zip for my intuition.




FWIW: A trick that helped me once with an old scanner driver was to run Windows XP on Virtualbox on my Mac. The old software got its hardware access again, just like it used to ten years earlier. The virtues of virtualization…
Yes, I could try that, but I think life’s too short, and the Betterlight was getting to be something that was more trouble than it was worth now that we have such great area capture devices. I think there’s a Cambo Actus in my future.
Love that 120mm AM ED. From 5:1 to 5:1 it can’t be beat. Also suprisingly resistant to flare. Supposedly at 1:1 its symetrical design completely eliminates lateral chromatic aberration.
Sorry for commenting on an ancient post, but I’m trying to get the math right on this myself and this post came up in searching. As careful as I know you are with your tests, I’m very suspicious of the result presented and am fairly confident your original intuition was correct – because it matches the math as well. That seems to suggest a flaw in the test.
First, we know that at a reproduction ratio of zero (i.e. infinity focus) the focal length of the lens will be irrelevant to the impact of a given tilt. In that case it would be a pure image plane problem, the image plane will be tilted exactly the same regardless of the focal length and the CoC in the image plane is independent of the focal length.
So that would imply somehow the “rules” are different at reproduction ratios such as your test (appears around maybe 0.2 to 0.4). But in fact they are not, in fact it is pretty foundational in the Scheimpflug principle, which was probably driving your intuition. See equations here:
https://en.wikipedia.org/wiki/Scheimpflug_principle#Depth_of_field
Note especially:
yx = (J * f * u’) / (uh * v’)
For your test setup which identical reproduction ratios u’/v’ is the same in both cases. In addition, for your setup J is proportional to f with the proportionality constant being the same for both lens setups. uh is proportional to f*f. Thus (J*f)/uh is also the same in both cases. Thus yx should be the same for both images.
They obviously aren’t in your test, which would somehow indicate Scheimpflug is wrong which would be pretty surprising.
There does, however, seem to be a pretty simple explanation. Is it possible that after fighting with the digital back that when you took the shots of the ground glass for the 65mm you didn’t use the T or B shutter setting to shoot at the set aperture on the shutter but instead used the manual shutter lever for previewing? I believe on at least some versions of the Copal 0 that when you use that manual lever that the aperture fully opens rather than only opening to the shooting aperture. You actually have to use T or B to get it to open only to the shooting aperture.
Note also that the difference in the DoF between the two shots appears to be quite close to a ratio of 1.4 (this by measuring the “height” of sharp focus in the two images, equivalent to the parameter y in the equations above). That matches the difference between your intended shooting aperture of F/5.6 and what the 65mm would have opened to using the manual lever on the shutter (F/4).
So it seems the math says they should be identical, and certainly gives no motivation for a difference of a factor of 1.4. An common error in using the shutter controls when directly viewing on ground glass would instead give the empirical result of a 1.4x change in DoF.
I know this thing is like five years old, and I can’t remember things I did five weeks ago, but maybe this is worth revisiting.